9.1 Worked Examples

In this example, a highway agency in the Northern U.S., labeled “The Northern Agency,” is interested in calculating asset value and related measures to report for highway-related assets in its TAMP. Note, this example is adapted from tests with two different agencies, and is not intended to be representative of any actual agency.

Following the process outlined in Section 2, the agency first establishes that its goal is to establish overall value and related measures for three asset classes: pavement; structures (including bridges and bridge-length culverts); and buildings. The agency has data at the asset-level for each asset class. For pavement and structures, the agency has detailed condition data. For buildings, the agency has only summary inventory data, but its facility division has separately established insurance values representing the amount each building is insured for in the event of a catastrophic event, independent of the value of land or the equipment in each building.

For their structures, the agency decides that asset value should be computed at a component level, given that different structure components have different useful lives and condition data are available to support the calculation. Bridges are represented using three components: the bridge deck, superstructure and substructure. Bridge-length culverts are represented as a single component.

The following subsections describe the approach used for Steps 2 to 4 of the asset value calculation by asset class, followed by a summary of the results.

Pavement

Using the flow chart in Chapter 4 the agency decides that initial value for pavement should be based on replacement cost, given there is no need to maintain consistency with the approach used for financial reporting (based on historic costs), no specific need to calculate value of the asset class to society (which would suggest a need for calculating economic value), nor is there a market value that may be readily determined as an alternative.

Next, the agency reviews its treatment strategy for pavements. Initial construction of pavement is estimated to cost $1.4 million per lane mile. When a pavement section reaches the end of its useful life it is reconstructed at a cost of approximately $1 million per lane mile, restoring it to “like new” condition. Various treatments are performed over a pavement’s life, and their effects are reflected in the Pavement Condition Index (PCI) at any given time. PCI is an agency-specific measure of pavement condition. It  combines different pavement distresses into a scale from 0% (worst condition) to 100% (best condition).

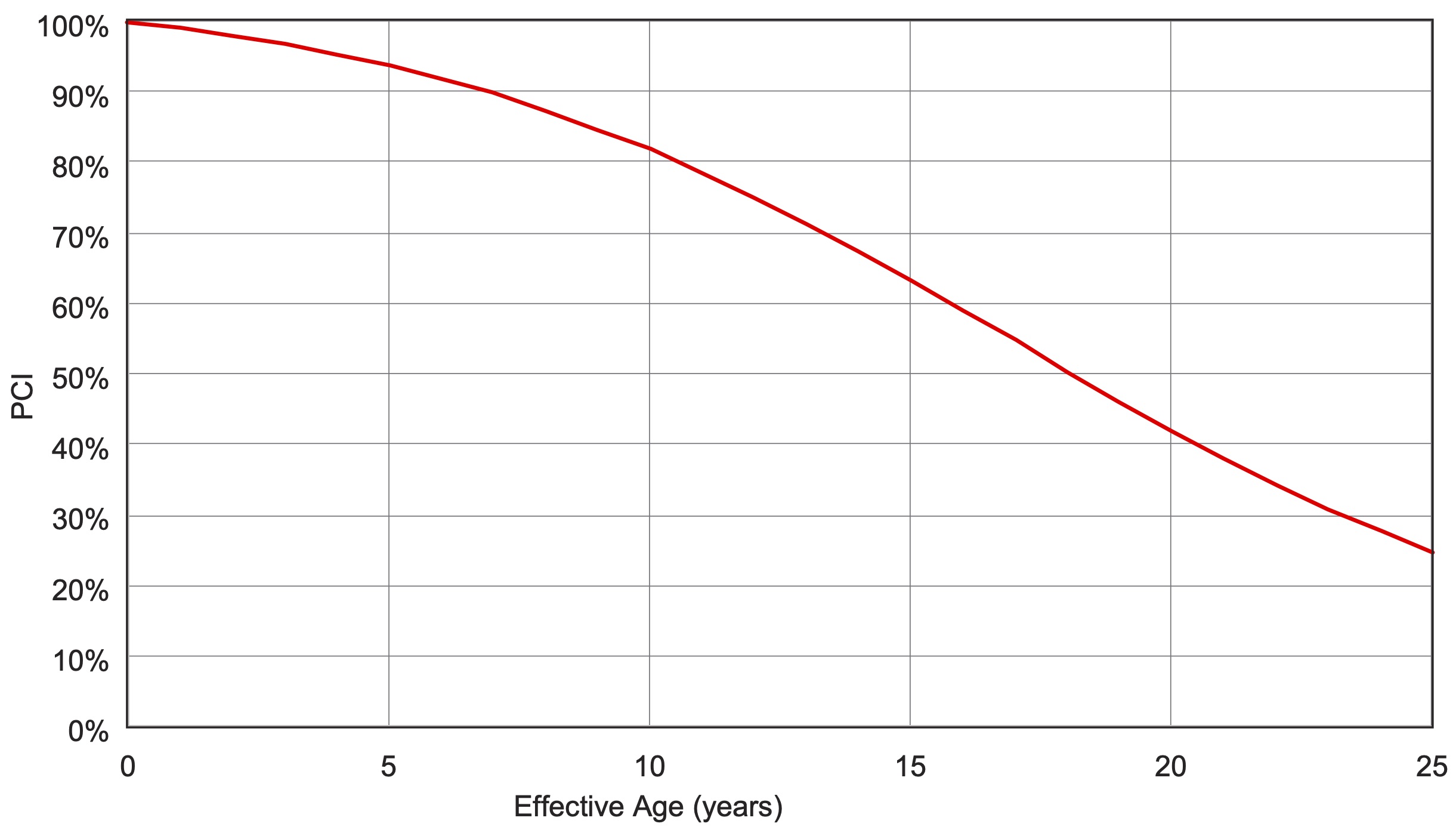
Given their use of the replacement cost approach to calculate initial value and PCI to capture condition, the agency determines it is not necessary to incorporate other treatments in the calculation of asset value besides pavement construction and reconstruction. Based on the agency’s life cycle strategy, the pavement is deemed to reach the end of its useful life when its PCI is reaches a value of 25%, which typically occurs at an age of approximately 25 years, as depicted in the deterioration curve shown in Figure 9-2. The pavement assets’ residual value is estimated to be $0.4 million per lane mile, equal to the difference in cost between initial construction and reconstruction.

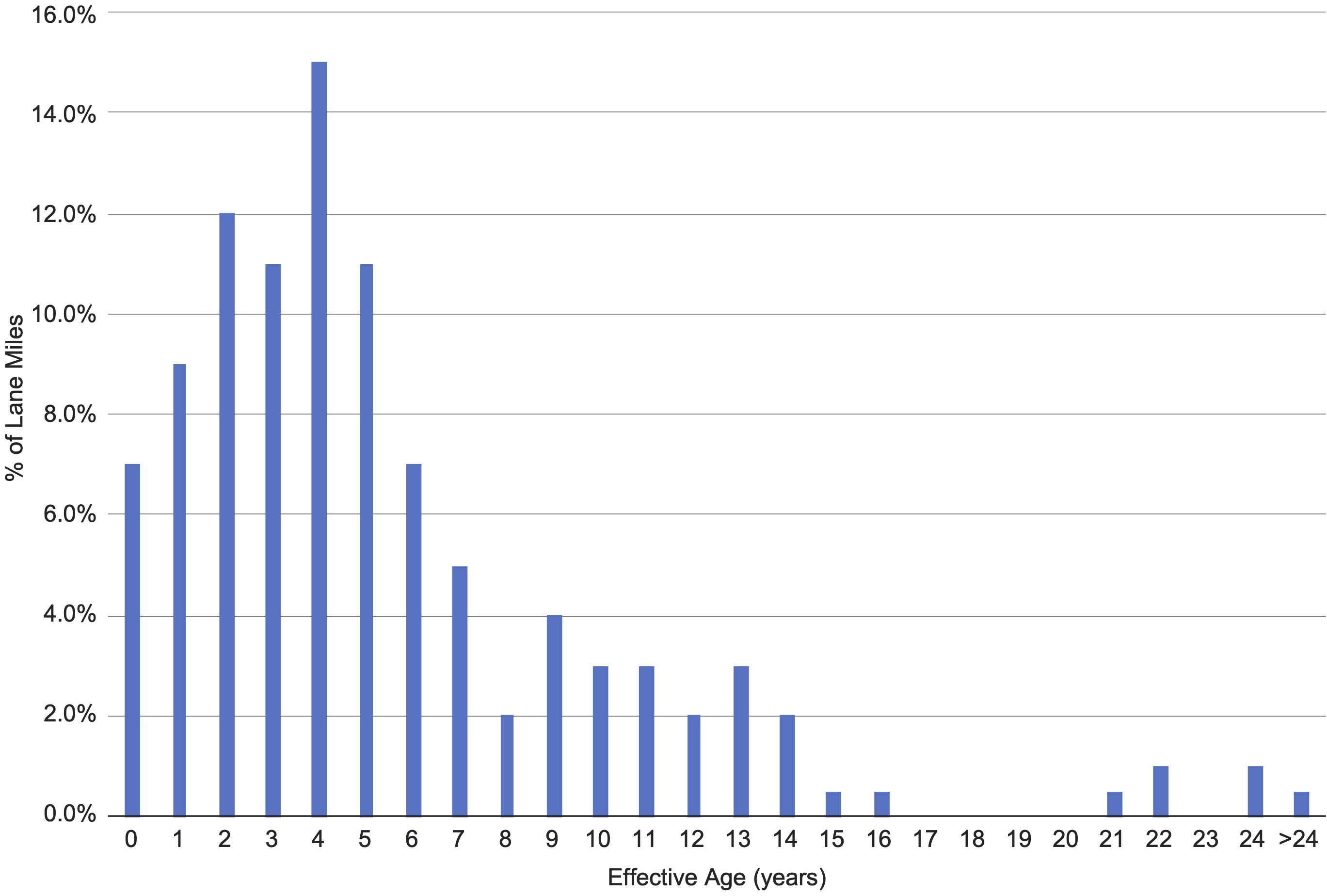
Then, the agency considers how to calculate depreciation. Reviewing the flow chart in Chapter 6, the agency decides to use a condition-based approach for calculating depreciation. The PCI of a pavement section estimates the effective life remaining where condition data are available. Where data are unavailable, the asset’s actual age is used. Figure 9-3 summarizes the distribution of pavement condition, depicting the percentage of pavement lane miles for each effective age from zero to over 24. Most of the assets have an effective age of under five years.

The agency uses the above information to calculate the value of its pavement. Initial value is approximately $30.8 billion (22,000 lane miles x $1.4 million per lane mile). For each section, depreciation is calculated based on the effective age using the depreciation formula for the condition-based approach, provided in Chapter 6. The result is that current pavement value is calculated as $26.0 billion with annual depreciation equal to $876 million. Annual depreciation is calculated by aging each pavement section by an additional year and noting the resulting change in value.

Structures

After completing the pavement valuation, the agency walks through the same process outlined above for structures, considering each of the bridge components defined. As in the case of pavement, the agency decides that initial value should be based on replacement cost.

The agency next reviews its treatment strategy for bridges and bridge length culverts. The construction of a bridge or culvert costs approximately $280 per square foot of deck area. Replacing a structure has a similar cost. The approximate costs for replacing bridge decks, superstructures and substructures are established based on a pro-rated share of the total bridge replacement cost, considering the relative costs of replacing the component parts. Based on these historic costs, the bridge deck replacement is estimated to be 25% of the value of the bridge, the superstructure is estimated to be 40% of the value, and the substructure is estimated to be 35% of the value. Various treatments are performed over the life of a component, and their effects are reflected in the component conditions ratings. These are measured on the 0 to 9 scale defined for the NBI. When a component has reached the end of its useful life either the component is replaced or the entire structure may be replaced.

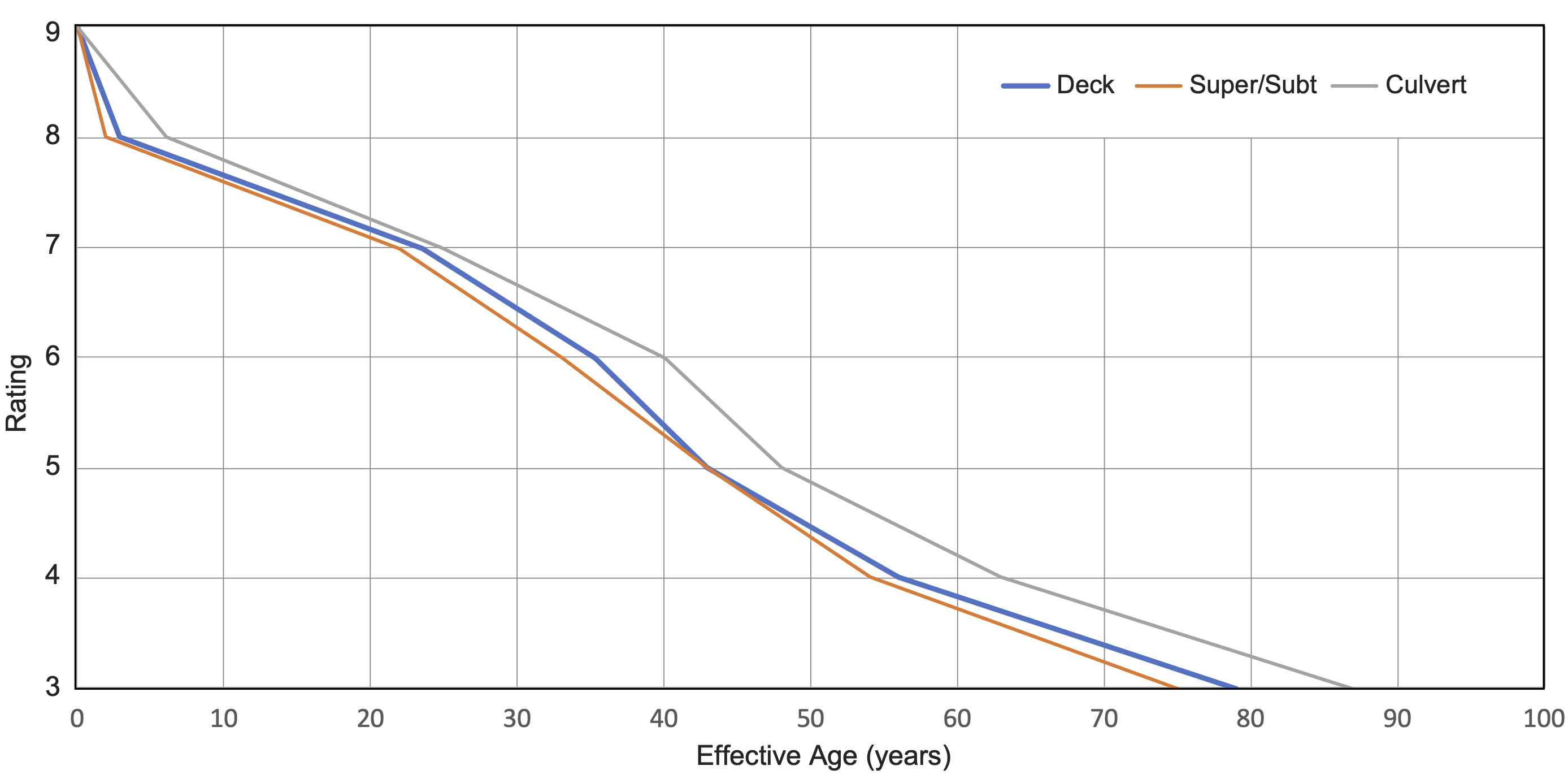
Given the incorporation of the component condition ratings into the NBI, the agency determines it is not necessary to include any treatments in the calculation of asset value besides initial construction and component or bridge replacement. Based on the agency’s life cycle strategy, a bridge deck is deemed to be at the end of its useful life when it has a rating of 4 or less on the NBI scale. For the superstructure, substructure and culverts, the component is deemed to be at the end of its useful life when it has a rating of 3 or less. Figure 9-4 illustrates the agency’s deterioration curves depicting the effective age of a component as a function of its condition rating. The agency developed these curves for use in their management systems based on an analysis of historic bridge inspection data. Three curves are shown in the figure: one for decks; a second used for both superstructure and substructure; and a third curve for culverts.

The agency further establishes that a portion of its bridges are built to outdated functional standards for load capacity and clearances. These bridges are deemed to be at the end of their useful life regardless of their physical condition. Thus, these bridges are treated as fully depreciated when calculating their asset value.

The agency then considers how to calculate depreciation. As in the case of pavement assets, the agency decides to use a condition-based approach. The NBI component rating is used to establish effective age. Figure 9-5 summarizes the distribution of conditions, depicting the percentage of each component in each condition rating (exempting functionally obsolete structures).

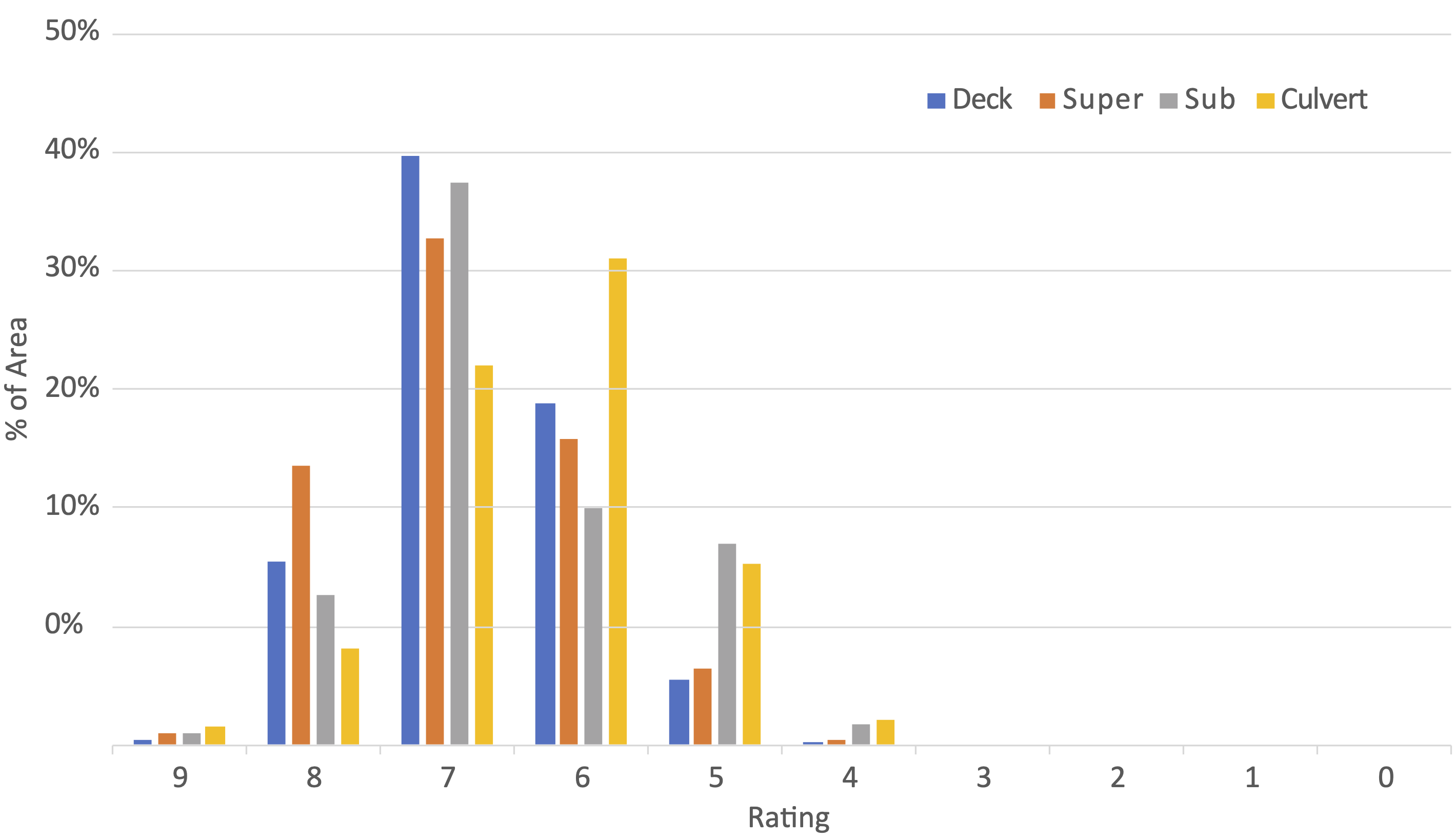
Lastly, the agency uses the approach described above to calculate value. Initial value is approximately $14.0 billion (50 million square feet x $280 per square foot). The calculations of current value are made by component, grouping all of the components of a given rating together (and exempting the obsolete bridges). For each rating group, effective age is estimated using the agency’s deterioration curves, and then depreciation is calculated based using the depreciation formula provided for the condition-based approach in Chapter 6. The result is a current structure value of $8.8 billion.  With annual depreciation equal to $193 million; this can be calculated by aging each group by an additional year and noting the resulting change in value.

Buildings

For its buildings, the agency has more limited condition data than it has for pavement and structures. However, as noted above, in addition to its data on the building inventory, the agency has data on the insured value of each of its buildings. The agency decides to use this insured value as a proxy for market value. The agency thereby establishes that the insured values of its buildings totals $0.9 billion.

The agency establishes the cost to replace all of its buildings would be approximately $1.2 billion, and that buildings are estimated to have a useful life of 60 years. The agency determines that an age-based approach should be used for depreciation if it is necessary to further depreciate the market value. Annual depreciation is estimated at approximately $20 million (equal to the replacement cost of $1.2 billion divided by the useful life of 60 years).

Results Summary

Table 9-1 summarizes the results of the asset value calculation. For each asset class, it shows the replacement cost, current value, ACR, and annual depreciation. The total replacement cost for all of the Northern Agency’s highway assets totals $46 billion. Considering the effects of depreciation, the current value of the inventory is $35.7 billion, resulting in an ACR of 0.78. Note there is no specific target value for ACR, but ideally this measure is maintained or increased over time. Absent investment to increase asset value, the inventory is expected to depreciate approximately $1.1 billion per year. This estimate of annual depreciation can be used as an estimate of the Cost to Maintain Value, though as described in Chapter 7 this cost should ideally be calculated using an agency’s asset management systems.

Table 9-1. Summary Results for the Northern Agency

Asset Class	Subclass	Replacement Cost ($ billion)	Current Value ($ billion)	Asset Consumption Ratio (ACR)	Annual Depreciation
Pavement		30.8	26.0	0.84	876
Structures	Bridges	13.4	8.5	0.63	187
	Culverts	0.6	0.3	0.61	6
	Subtotal	14.0	8.8	0.63	193
Buildings		1.2	0.9	0.75	20
Total		46.0	35.7	0.78	1,089

This example presents a transit agency, located in the Western U.S., termed “The Western Agency.” The agency operates three different transit modes: buses; paratransit vehicles (also called “cutaways”); and a Light Rail Transit (LRT) system. The agency’s asset hierarchy is summarized in Figure 9-6. Major asset classes include revenue vehicles, equipment (service vehicles), facilities and infrastructure. Each of these asset classes consists multiple subclasses. The infrastructure asset class includes the largest number of subclasses. In addition to LRT track, which may be either tangent (straight) or curved, this class includes bridges, special trackwork (grade crossings and switches), and power assets (catenary wire segments, relay cases, and substations).

Previously the Western Agency reported asset value in its TAMP based on the historic cost of asset acquisition or construction. This approach was used to maintain consistency with the agency’s reporting of asset value in its financial statements. As part of the previous effort, the agency collected data on the historic cost and year of purchase or construction for each of the asset classes and subclasses in the TAMP. For revenue vehicles, equipment and facilities costs are tracked at the asset level. For infrastructure assets, costs are tracked by asset subclass, grouping all assets of a given subclass built at a similar time.

For its next TAMP, the agency seeks to report value based on current replacement cost rather than historic cost, as current replacement cost is more closely tied to the cost of rehabilitating and replacing assets. However, for now the agency intends to include both the historic cost of its assets and the current depreciated replacement cost to facilitate comparison with the values in its prior TAMP.

The following subsections describe the approach the agency used for the asset value calculation, followed by a summary of the results. The asset classes are combined into two groups in the discussion: vehicles, which includes revenue vehicle and equipment; and fixed assets, including facilities and infrastructure.

Revenue Vehicles and Equipment (Service Vehicles)

As described above, the agency seeks to establish initial value based on current replacement cost. The agency estimates this initial value by adjusting the historic cost paid for each vehicle for inflation. The agency estimates the annual inflation rate for revenue and service vehicles has historically been approximately 1.6 percent.

The agency next reviews its treatment strategy for vehicles. The agency has established the useful life for its vehicles by vehicle type assuming that planned maintenance and rehabilitation activities are performed on a vehicle over its useful life. The useful life shown in Table 9-2 is estimated based on historical asset performance. At the end of a vehicle’s useful life the vehicle is replaced with a new vehicle. Since vehicle treatments are included within the useful life estimates, the agency establishes that the only treatment explicitly modeled in the asset value calculation should be the initial purchase of the vehicle. While the agency auctions used assets at the end of their useful life, the value received is negligible, so for the sake of this analysis, they assume a residual value of $0. To depreciate vehicle asset value the agency elects to use an age-based approach. The depreciation calculation is made separately for each vehicle.

Table 9-2. Useful Life for Vehicles

Asset Class	Subclass	Useful Life (years)
Revenue Vehicles	Buses	14
	Light Rail Vehicles	40
	Paratransit Vehicles	10
Equipment (Service Vehicles)	Automobiles	8
	Steel Wheeled Vehicles	25
	Trucks and Other Rubber-Tired Vehicles	14

Facilities and Infrastructure

As in the case of vehicles, the agency seeks to establish initial value based on current replacement cost. The agency estimates this initial value by adjusting the historic construction costs of each asset for inflation. The agency estimates the annual inflation rate for construction has been approximately 3.0 percent over the facilities’ lifespan.

Next, the agency reviews its treatment strategies for fixed assets. For these assets, the agency periodically measures asset conditions using the five-point condition scale established by FTA. Using this scale, condition ranges from 1 (worst condition) to 5 (best condition). If an asset has a condition of 2 or less it is deemed to be not in good repair and beyond its useful life. Useful lives are established by asset class, as shown in Table 9-3. When an asset has reached the end of its useful life it is replaced. The agency performs different treatments on an individual asset over its life, but these are reflected in its condition score. Given the availability of condition data, the agency establishes that the only treatment explicitly modeled in the asset value calculation is the asset construction. The agency further assumes that the residual value of an asset at the end of its life is $0.

Table 9-3. Useful Life for Fixed Assets

Asset Class	Subclass	Useful Life (years)
Facilities	Administrative	60
	Maintenance
	Public Facilities
Infrastructure	LRT Track - Tangent	35
	LRT Track - Curved	30
	LRT Bridges	70
	Grade Crossings	15
	Switches	25
	Catenary Wire Segments	25
	Relay Cases	50
	Substations	25

To depreciate asset value the agency elects to use condition-based approach assuming that the change in condition is proportional to age. The effective age of an asset as a function of condition is modeled as follows:

\begin{align*} E(c) &= \frac{UL (5-c)}{(5-2)} \end{align*}

where E(c) is the useful life for a given condition c and UL is the useful life. The denominator is represented by the condition rating at the end of the asset’s useful life subtracted from the highest possible condition rating. With this approach, the effective life of an asset is 0 if its condition is rated as a 5 and is equal to its total useful life if the rating is 2 and it is fully depreciated.

Results Summary

Table 9-4 summarizes the results of the asset value calculation. For each asset class and subclass, it displays the historic cost, replacement cost, current value, ACR and annual depreciation. The table shows that the historic cost of the agency’s assets is $3.1 billion. Adjusting the historic costs for inflation, the current replacement cost of the asset inventory is estimated to be approximately $4.5 billion. The current value, which incorporates depreciation, is approximately $2.7 billion. The ACR for the agency’s asset inventory is 0.60, equal to the current value divided by the replacement cost. Absent investment in the assets, the inventory is expected to depreciate approximately $123 million per year.

Table 9-4. Summary Results for the Western Agency

Asset Class	Asset Subclass	Historic Cost ($M)	Replacement Cost ($M)	Current Value ($M)	Annual Depreciation ($M)	ACR
Revenue Vehicles	Bus	456	498	298	34	0.60
	Light Rail Vehicle	506	603	433	15	0.72
	Paratransit	25	26	17	3	0.65
	Subtotal	987	1,127	748	51	0.66
Equipment	Automobiles	3	3	1	0	0.27
	Steel Wheeled Vehicles	2	2	2	0	0.86
	Trucks and Other Rubber-Tired Vehicles	35	39	23	3	0.60
	Subtotal	40	44	26	3	0.59
Facilities	Administrative	19	39	23	1	0.58
	Maintenance	170	400	195	7	0.49
	Public Facilities	925	1,485	789	25	0.53
	Subtotal	1,114	1,924	1,007	32	0.52
Infrastructure	LRT Track - Tangent	503	710	473	20	0.67
	LRT Track - Curved	144	203	135	7	0.67
	LRT Bridges	190	268	161	3	0.60
	Grade Crossings	4	6	3	0	0.57
	Switches	4	5	3	0	0.50
	Catenary Wire Segments	35	50	26	2	0.53
	Relay Cases	65	87	70	2	0.80
	Substations	34	46	28	2	0.60
	Subtotal	980	1,374	899	37	0.65
Total		3,121	4,469	2,680	123	0.60

In this example, a state department of transportation in the Midwest, labeled the “Midwest DOT,” applies the economic value approach to quantify benefits realized by users of the state’s primary roadway network. For this example, the state’s primary roadway network is defined as all state-owned Interstates, primary arterials, minor arterials, and major collectors. This example follows the economic approach described in Chapter 4 to estimate the benefits of the primary roadway system. The example provides a better understanding of the value generated by the roadways for direct users and society as a whole. This example shows how state DOTs can estimate the value assets provide to users as compared to the replacement value method, which focuses on what those assets cost.

Application of Economic Value Approach

This case study includes the following basic steps:

• First, traffic data were collected on the Midwest DOT’s primary roadway network. This included all Interstates, principal arterials, minor arterials, and major collectors.
• Second, fatality and serious injury crash rates were collected. These data are maintained by the Midwest DOT and available at the state level.
• Third, emissions rates for the state were collected from the Environmental Protection Agency (EPA) MOVES model. A representative county was selected to generate emissions rates specific to the state.
• Fourth, these data were used to calculate user benefits for the value of travel time, vehicle operating costs, safety, and emissions.
• Fifth, the research team examined the model to interpret the results and found that users of the roadway network experience a much higher monetary value of benefits than the Midwest DOT reports in its TAMP as the replacement costs of the system.

These steps are detailed in the following subsections. A theoretical discussion of the approach is presented at the end of the case study.

Data Collection

The Midwest DOT reports traffic data annually to the Federal Highway Administration (FHWA) as part of its Highway Performance Monitoring System (HPMS) reporting requirements. This data provides a convenient summary of traffic data that can be collected for any state DOT. While 2020 HPMS data was available, this example referenced 2019 HPMS data to avoid any distortions due to restrictions and changed habits during the COVID-19 pandemic. This is consistent with 2021 USDOT guidance for federal discretionary grants, which noted that 2019 traffic data should be used rather than non-representative 2020 data (48).

The analysis utilized the following HPMS variables that were provided by the Midwest DOT:

• Route_ID – This variable is assigned to each individual roadway segment. This identification number is alpha-numeric and contains information on roadway direction, type, and location.
• Section_Length – This variable refers to the length, in miles, of each identified roadway segment. This variable was used, in part, to calculate vehicle-miles traveled (VMT) and vehicle-hours traveled (VHT). These two variables were the basis for estimating all benefits categories included in the analysis.
• F_System – This variable indicates the classification of the system and is broken into seven different types. Segments classified as 1, 3, 4, and 5 were isolated as those correspond with Interstates, principal arterials (other), minor arterials, and major collectors, respectively. Roadways classified as 2, 6 and 7—principal arterials (other freeways and expressways), minor collectors, and local roads, respectively—were not included in the analysis because they are not part of the principal roadway system and did not have a significant number of observations in the dataset.
• Facility_Type – This variable refers to the operational characteristic of the roadway. This variable is important to account for the correct mileage and average annual daily traffic (AADT). Most Interstate segments and many principle arterial segments are separated directionally. The codes used for this variable are as follow:
  • 1 – One-way roadway
  • 2 – Two-way roadway
  • 3 – Couplet
  • 4 – Ramp
  • 5 – Non-mainline
  • 6 – Non-inventory direction
  • 7 – Planned/unbuilt

In the HPMS database, many separated Interstates and principal arterials are reported as bidirectional AADT for each separated direction of roadway, so the same bidirectional AADT is reported as Facility_Type 1 in the eastbound direction of a roadway and as Facility_Type 6 in the westbound direction. In order to avoid double-counting of AADT, roadway segments classified as “Non-inventory direction,” or Facility_Type 6, were excluded from this analysis.

• Ownership – This variable indicates the entity that has legal ownership of a roadway and is typically used for apportionment, administrative, legislative, analytical, and national highway database purposes and in cost allocation studies. This example only considers segments with Ownership code 1, which refers to roadways owned by the State DOT.
• Urban_Codes – This variable refers to the U.S. Census Urban Area Code and is used for the querying and analyzing data by the unique identification of a state’s urbanized areas and generically by small urban or rural areas. For this example, the values of urban and rural travel are separated. All segments coded as 99998 or 99999 were considered rural and all others were considered urban.
• AADT – This variable provides the bi-directional annual average daily traffic counts of all vehicles and trucks traveling on the roadway. This variable was used, in part, to calculate VMT and VHT. As discussed above, the “Facility_Type” variable was used in combination with AADT to ensure that bi-directional AADT is not “double counted” in the case of divided or separated roadways.
• AADT_Combination – This variable provides the bi-directional annual average daily traffic counts for combination trucks. Trucks have different operating costs, emission rates, and values of time, so these vehicles are treated separately from passenger vehicles in the analysis. This variable was used, in part, to calculate VMT and VHT as discussed further below.
• AADT_Single_Unit – This variable provides the bi-directional annual average daily traffic counts for single-unit trucks. Trucks have different operating costs and emission rates, and their value of time is calculated differently, so these vehicles are treated separately from passenger vehicles in this analysis. This variable was used, in part, to calculate VMT and VHT.
• Speed Limit – This variable reports the posted speed limit for every roadway segment. The Midwest DOT advised that due to negligible congestion statewide, the research team should use this as the measure for speed of travel. This variable was used, in part, to calculate the VHT of vehicles.

To summarize, roadway segments were sorted to include only those that are Midwest DOT-owned and classified as F_System type Interstate, principal arterial, minor arterial, or major collector. This calculation did not include minor collectors and local roads. Further, segments identified as non-inventory direction under the Facility_Type variable were excluded to prevent any double counting of AADT.

For each F_System type, VMT was calculated separately for urban and rural areas as identified by their Urban Codes. VMT was also calculated separately for passenger vehicles and trucks and aggregated along the criteria stated. The basic calculations for VMT are found below. Note that these calculations represent the VMT calculation for each individual segment. Total VMT is the sum of VMT for all roadway segments, calculated for Interstates, principal arterials, minor arterials, and major collectors and separately for urban and rural areas.

• Passenger Vehicle VMT = [Total Vehicles (AADT) – Total Trucks (AADT_Combination + AADT_Single_Unit)] * Section Length
• Truck VMT = [Total Trucks (AADT_Combination + AADT_Single_Unit)] * Section Length

VMT and speed limits were used to calculate VHT for the same roadway segment criteria. Total passenger vehicle and truck VHT were calculated by summing the VHT from each roadway segment. To calculate VHT, passenger vehicle and truck VMT for each segment was divided by the posted speed limit (in miles-per-hour), which was used as a proxy for average travel speed for this exercise. Typically, observed average travel speeds or VHT collected by the state or calculated using traffic modeling software would be preferable as a measure of vehicle speed, but in this instance the relevant roadways had sufficiently low to nonexistent congestion levels, so speed limit was used as an acceptable measure. The general calculation for passenger vehicle and truck VHT can be found below. Note that this is the VHT calculation for each individual segment. Total VHT would be the summed total of all the roadway segments.

• Passenger Vehicle VHT = Segment Passenger Vehicle VMT / Segment Speed Limit
• Truck VHT = Segment Truck VMT / Segment Speed Limit

User Cost Calculation

In the next step, monetary values were applied to the VMT and VHT aggregations to calculate the user benefits (or in this case, user costs) associated with willingness-to-pay for travel time, vehicle operating costs, safety, and emissions. This analysis utilized monetization parameters recommended per the United States Department of Transportation (USDOT) Benefit-Cost Analysis Guidance for Discretionary Grant Programs as of February 2021 (48).

Travel Time

USDOT guidance recommends a value of time for passengers and truck drivers of $17.90 and $30.80 per hour, respectively. Passenger vehicles are assumed to have 1.67 occupants and trucks are assumed to have one occupant. The basic calculations for the value of travel time for passenger vehicles and trucks used in this analysis can be found below:

• Total Value of Travel Time for Passenger Vehicles = $17.90 * 1.67 occupants * Total Passenger Vehicle VHT
• Total Value of Travel Time for Trucks = $30.80 * 1 occupant * Total Truck VHT

Vehicle Operating Cost

USDOT guidance recommends a per-mile vehicle operating cost of $0.43 for passenger vehicles and $0.93 for trucks. These values were applied directly to the VMT calculated for passenger vehicles and trucks. The basic calculations for the value of vehicle operating costs used in this analysis can be found below:

• Total Value of Passenger Vehicle Operating Costs = Total Passenger Vehicle VMT * $0.43
• Total Value of Truck Operating Costs = Total Truck VMT * $0.93

Safety

USDOT guidance recommends a monetized value of an averted fatality of $10,900,000 and a monetized value of an averted injury (of unknown severity) of $197,600. The Midwest DOT provided crash rates by severity and facility type for rural and urban areas in its Highway Safety Improvement Program (HSIP). These rates are reported as incident rates per hundred-million vehicle miles traveled (HMVMT). The basic calculations for the value of safety incidents used in this analysis can be found below:

Fatality Crashes

• Total Value of Passenger Vehicle Fatal Crashes = [Total Passenger Vehicle VMT / 10^{^6}] * $10,900,000 * Fatal crash rate per HMVMT
• Total Value of Truck Fatal Crashes = [Total Truck VMT / 10^{^6}] * $10,900,000 * Fatal crash rate per HMVMT

Injury Crashes

• Total Value of Passenger Vehicle Injury Crashes = [Total Passenger Vehicle VMT / 10^{^6}] * $197,600 * Injury crash rate per HMVMT
• Total Value of Truck Injury Crashes = [Total Truck VMT / 10^{^6}] * $197,600 * Injury crash rate per HMVMT

Emissions

USDOT recommends emissions valuations per metric ton of pollutant emitted by pollutant type and year. These monetized values are applied to pollutant quantities calculated using the EPA MOVES model for a representative county in the state. The MOVES model reports grams of pollutant emitted per mile driven. It has separate emissions rates for passenger vehicles and trucks, urban and rural roadways, and various speed bins which consider the different fuel efficiency experienced at different speeds. Below are the monetized values per metric ton of pollutant emitted by type and year.

Table 9-5. Cost per Metric Ton of Pollutant from the MOVES Model

Emission Type	NOx	SO2	PM2.5	CD2
2020	$15,700	$40,400	$729,300	$50
2021	$15,900	$41,300	$742,300	$52
2022	$16,100	$42,100	$755,500	$53
2023	$16,400	$43,000	$769,000	$54
2024	$16,600	$43,900	$782,700	$55
...	...	...	...	...
2050	$18,000	$48,200	$852,700	$84

The basic calculation for the value of emissions used in this analysis can be found below:

• Total Value of Passenger Vehicle Emissions = Total Passenger Vehicle VMT * [Grams of Pollutant Emitted by Type / 1000^{^2}] * Monetary Value of Pollutant by Type
• Total Value of Truck Emissions = Total Truck VMT * [Grams of Pollutant Emitted by Type / 1000^{^2}] * Monetary Value of Pollutant by Type

The value of emissions is dependent on the year in the analysis so annual emissions should be calculated for each year and applied to that specific year’s monetary value.

Analysis Period and Discounting

This exercise used a 20-year analysis period, which is consistent with USDOT BCA guidance. The beginning year for values was set at 2020 and concluded in 2039 to cover a 20-year period. In accordance with USDOT guidance, all monetized values were discounted at 7 percent with the exception of values related to carbon dioxide emissions, which were discounted at 3 percent. The general formula for calculating the discount rate can be found below:

• Discount Rate = 1 / [(1 + Discount Rate) ^{^ (Year of Analysis – Base Year of Analysis)}]

Discounted benefits for each year were aggregated to calculate the discounted total benefits realized by the state’s primary roadway network over the analysis period. Because the benefits were calculated separately by F_System type and for urban and rural areas the total benefits can be viewed with added levels of granularity as needed.

Results of Economic Value Approach

This example reveals significant value in the state’s primary roadway network. Annual travel recorded in 2019 was projected with no assumed growth rate for each year of the analysis period. The value experienced by roadway users in Year 1 of the analysis was calculated at $13.2 billion. Over a 20-year time period this equates to $258.7 billion in undiscounted terms or $148.0 billion when discounted at 7 percent (3 percent for carbon dioxide emissions). The most significant drivers of value were travel time and vehicle operating costs, which were $5.7 billion and $6.1 billion (annually), respectively.

Table 9-6. Summary of the Midwest Agency’s Roadway Network Value

User Cost Category	Single-Year Value ($B)	20-Year Value Undiscounted ($B)	20-Year Value Discounted at 7% (3% for CO2) ($B)
Travel Time	$5.74	$114.79	$65.06
Vehicle Operating Costs	$6.05	$121.02	$68.59
Traffic Safety	$0.71	$14.10	$7.99
Emissions	$0.66	$8.75	$6.31
Total	$13.16	$258.66	$147.95

The single year value experienced by the roadway is roughly half of the network’s total replacement value as reported in the Midwest DOT’s Transportation Asset Management Plan. This implies that after approximately two years of use the roadway network has already provided value to the public equal to the entire replacement cost of the network. A significant portion of the state’s travel occurs on the primary network and this is reflected in the significant value experienced by users.

Overall, the value realized in urban areas ($7.1 billion annually) is slightly greater than that realized in rural areas ($6.1 billion annually).

Table 9-7. Single Year Value for Urban and Rural Roads at the Midwest Agency.

User Cost Category	Urban Single-Year Value ($B)	Rural Single-Year Value ($B)	Total Single-Year Value ($B)
Travel Time	$3.39	$2.35	$5.74
Vehicle Operating Costs	$3.00	$3.05	$6.05
Traffic Safety	$0.41	$0.30	$0.71
Emissions	$0.25	$0.41	$0.66
Total	$7.06	$6.10	$13.16

At the state level, Interstates account for $9.9 billion in annual value, principal arterials account for $3.2 billion, minor arterials account for $0.04 billion, and major collectors account for $0.02 billion. For the Interstate system alone, the rural annual value ($6.05 billion) exceeds urban annual value ($3.8 billion). Approximately 99 percent of the total value of Midwest DOT rural roadways is on the Interstates, with only $45 million of rural annual value coming from principal arterials, minor arterials, and major collectors. Principal arterials represent a much larger share of value in urban areas.

Overall, Interstates account for 75.0 percent of value, principal arterials account for 24.5 percent, and combined minor arterials and major collectors account for the remaining 0.5 percent.

Table 9-8. Single Year Value for Each Road Category at the Midwest Agency.

User Cost Category	Interstate Single-Year Value ($B)	Principal Arterial Single-Year Value ($B)	Minor Arterial Single-Year Value ($B)	Major Collector Single-Year Value ($B)	Total Single-Year Value ($B)
Travel Time	$4.05	$1.67	$0.02	$0.01	$5.74
Vehicle Operating Cost	$4.79	$1.24	$0.02	$0.01	$6.05
Traffic Safety	$0.47	$0.23	$0.01	$0.00	$0.71
Emissions	$0.57	$0.09	$0.00	$0.00	$0.66
Total	$9.87	$3.23	$0.04	$0.02	$13.16

It is clear that the Midwest DOT’s primary roadway network generates significant user value in the state. The value generated in a single year equates to roughly half the total replacement cost as reported in the Midwest DOT’s TAMP annually. This highlights the high value the Midwest DOT creates by simply maintaining the existing infrastructure. The service life of roadways extends well beyond the payback period and generates value many times over the replacement cost in both discounted and undiscounted terms. The primary roadway network is an important societal and economic asset in the state worth far more to its users than is captured by the replacement cost approach.

Theoretical Framework of Economic Approach Example

This example applied the economic value approach to assess the value of the Midwest DOT’s primary roadway network. The implementation of such a system-wide assessment is challenging to conduct using the economic value steps described in Chapter 4, because it is difficult in practice to identify what the “next best alternative road class” would be relative to the entire state-owned roadway network.

On a smaller scale, such as that of an individual roadway improvement project, economic value can be assessed by comparing projected user costs with and without the infrastructure (e.g., bridge or roadway segment) being considered. However, in a system-wide assessment (e.g., for the entire state highway network), the with and without project contexts cannot be evaluated because travel behavior would be fundamentally different without the highway system. Trying to run a travel demand model without the state highway system would produce extreme results that do not reflect how society or travel would operate without the state highway system.

Given these limitations, this example assesses economic value as the sum of all observed user costs incurred for travel along the Midwest DOT’s primary roadway network. The theoretical basis of this valuation exercise can be explained by considering the value of an individual trip. If an individual chooses to travel from point A to point B and incur all the associated costs of doing so, then the overall value of that trip to the individual must at least be equal to the total costs incurred by the individual over the course of the trip. If the trip costs more to the individual than it is worth, that persons will not make the trip. Put another way, an individual will choose to travel along infrastructure only when the value realized is greater than (or potentially equal to) the cost of travelling. Accordingly, one can calculate the minimum value of a trip by monetizing the cost of the trip.

This theoretical approach can be expanded beyond an individual trip and applied to all trips across a roadway network. The aggregate value to society of all travel on a roadway network must at least be equal to the sum of all user costs incurred for travel on that roadway network. These total user costs include vehicle operating costs, value of travel time, monetized emissions costs, and injury and fatality crash costs.

Observed User Costs versus Willingness-to-Pay

The theoretical framework that underlies this example is related to the economic concept of “willingness-to-pay.” If a user is willing to pay a maximum of x dollars for a good, then that good must be worth x dollars to the individual. If the individual has the opportunity to purchase the good for any amount up to x dollars then a rational person will make that purchase, but if the cost of the good is above x then a rational person will choose not to purchase the good.

While willingness-to-pay reflects value, this value can be difficult to measure in practice. Willingness-to-pay for any given good varies greatly across individuals, but the prices of goods are much more standardized. Accordingly, most market transactions end up being made at a price below maximum willingness-to-pay for any given purchaser. An individual may be willing to pay up to x dollars for a good, but the person is likely in practice to find a price below x dollars for the goods that purchase. This is the case in using the user costs to estimate the value of travel in the Midwest DOT example.

While the amount paid (or costs incurred) for travel can be measured using the data available in this example, total willingness-to-pay cannot be assessed. The available data can be used to calculate what users do pay for travel, but it cannot be used to show what users would be willing to pay if travel were more expensive. As total willingness-to-pay reflects value and amount paid must be no greater than, and is often less than, willingness-to-pay, it follows that total amount paid is an underestimate of total value. Thus, the observed cost of all travel on the state’s primary roadway network reflects a “floor”—or a conservative understatement—of the total value of travel, and the actual value of travel realized across the roadway network must exceed this cost on the societal level. The exact extent to which actual value exceeds aggregate user costs depends on the economic concept of “elasticity of demand” for travel, which cannot be assessed in this example.

Implications of Measuring Observed User Costs (versus Willingness-to-Pay)

Since user costs represent a minimum user value, the implication of the economic value approach is that a decrease in travel costs within the state could appear to represent a decrease in value of the Midwest DOT’s infrastructure. For example, a hypothetical improvement to roadway conditions that leads to faster travel times, lower emissions, or safer roadway conditions would result in lower per-user travel costs being borne by users. Under an assumption of relatively inelastic demand, this decreased per-user travel cost would also result in lower aggregate observed travel costs across the state. Using the economic value approach, this improvement in roadway conditions would apparently decrease the value of the roadway, as the lower aggregate travel cost would reflect an apparent lower economic value of infrastructure. This seemingly contradictory finding—infrastructure that is more beneficial to its users should have greater economic value, not less—is a result of the data limitations that restrict the analysis to measuring only the observed travel costs borne by users (which were reduced in this example) rather than their true willingness-to-pay for travel.

While a roadway condition improvement may decrease the cost of travel along a route, it does not decrease willingness-to-pay for that travel. Thus, the lower user costs resulting from the improvement project will widen the “gap” between willingness-to-pay and cost borne. Total value realized by users will not change for all those pre-existing users of the facility, and any additional users who choose to use the facility as a result of the improvement project will realize additional value. Thus, while the lower per-user cost may appear to reflect lower economic value of infrastructure, it really represents a more conservative estimate of user value, which remains unchanged.

Limitations

There are at least four additional limitations to the analysis conducted in this case study:

• Internalization of emissions costs and crash risk – This example included vehicle operating costs, value of travel time, monetized emissions costs, and injury and fatality crash costs as observed user costs of travel. However, emissions costs and safety risk may not be fully “internalized” by users. That is, do individuals who make choices to travel on roads fully appreciate the crash risk associated with their travel and do they fully bear the societal costs of the emissions from their vehicles? If these costs are not fully “internalized” by the travelers, then it may be inappropriate to consider those costs as a component of “willingness-to-pay” for travel.
• Fixed travel costs – This example considers the marginal cost per-mile and per-hour of travel but does not consider the fixed (or sunk) costs also required for travel. Annual vehicle ownership costs, for example, do not directly depend on distance traveled per year. Thus, monetizing the revealed marginal cost of travel on a per-mile or per-hour basis understates the total cost of travel on an annual basis, and accordingly understates the total willingness-to-pay for travel.
• Roadway maintenance expenses – An additional cost of travel borne by society is the cost of construction, maintenance, and upkeep of public infrastructure. Some, but not all, of this cost is incorporated in user expenses, such as gasoline taxes and tolls. To capture the observed user costs of travel fully, it would be necessary to account for all roadway construction, maintenance, and upkeep expenses. However, these expenses cannot fully be captured using available data and applied to per-mile or per-vehicle parameter estimates.
• Apportionment of the value of travel – A challenge in applying willingness-to-pay theory to the valuation of roadway infrastructure is apportioning the overall value of travel among different components necessary for travel. For example, travel along an Interstate requires the Interstate infrastructure, but it also requires a vehicle and a source of fuel. Interstate travel cannot happen if any one of these multiple required inputs is unavailable. It would therefore be inappropriate to apportion the total value of Interstate travel to the Interstate infrastructure itself, as this would leave no value for the vehicle and fuel that are also required for travel. The willingness-to-pay theory behind this example does not provide insight as to what percentage of the overall value of travel should be apportioned to the roadway itself, versus what should be apportioned to other inputs required for travel.